Rule of 72: Expertly Know When Your Money Doubles in Value

Do you want to quickly calculate how many years it will take you to double your investment based on its annual rate? Or the specific rate that would double your money in a given number of years?

Does that feel like something hard that needs a complex calculator or spreadsheet? Fear not. With the rule of 72, some quick mental math or a few taps on your phone, you can get those answers easily.

By the time you are done reading this, you will feel confident to calculate the years it will take for your money to double in value.

Alternatively, if you know the years required, you will learn how to determine the exact annual rate needed to double your money.

Let’s add this powerful tool to your personal finance arsenal.

Formula for Rule of 72

The formula involves dividing 72 by the rate of return of the asset you are investing in.

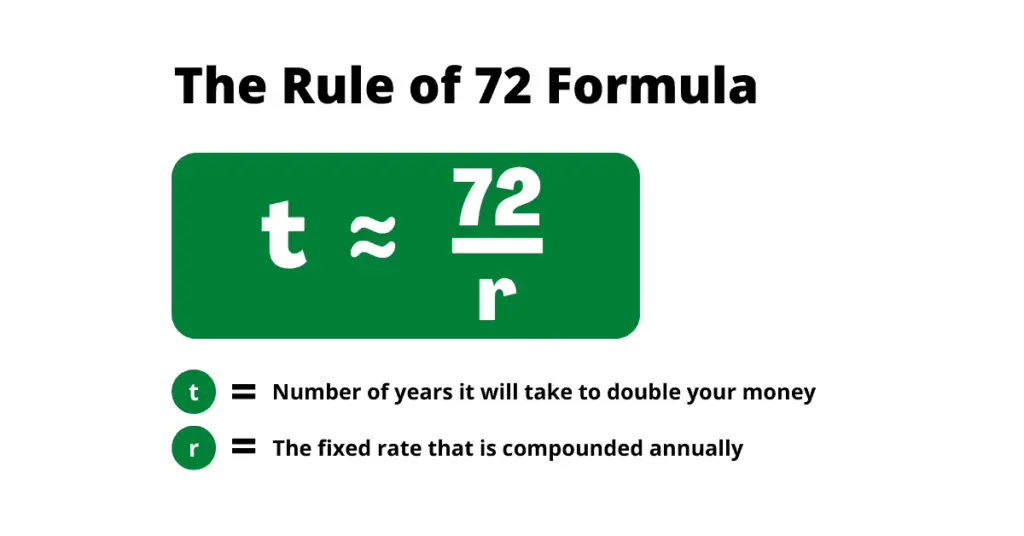
The answer to the formula above is the years that it will take for your money to double if it’s earning compound interest at a fixed rate.

Rule of 72 Investing

Supply:

• Rate of return
• Number of years investing

Tools:

• Basic calculator
• Mental math

Materials: Formula for rule of 72

Rule of 72 Finance

The future value of most financial products such as loans can balloon if one doesn’t pay the principal in time. This is due to the compounding interest charged by the loan issuer.

You can use the rule of 72 to assess the cost of not paying off your debt within the specified time periods.

72/10 = 7.2 years to double your current balance owed

For example, if the loan rate is 10%, then not paying the debt would result in the amount owed doubling every 7.2 years!

Rule of 72 Inflation – purchasing power losing half its value

The rule of 72 is an easy way to assess the impact of inflation on your purchasing power. For example, your purchasing power will lose half its value every 14.4 years at an average inflation rate of 5%!

Here is the calculation on how I arrived at the 14.4 years’ estimate.

72/5 = 14.4 years for your purchasing power to lose half its value

How does the Rule of 72 work

In this section, you will learn what works as per the rule of 72 definition. There are certain things to consider when using the rule.

Rule of 72 compound interest considerations

It works with compounding interest and not on simple interest calculation.

Compounding interest is where you earn interest on interest. For example with daily compounding, your principal would earn interest on the first day.

Day 1 Interest = Interest rate/365 * Initial value

On the second day, the interest earned on Day 1 plus the principal would form the new principal amount for Day 2.

Day 2 Interest = Interest rate/365 * (Initial amount + Day 1 interest)

The compound interest will continue to be earned until the end of the time period. Therefore the accumulated interest helps the initial amount to grow much faster!

In contrast, simple interest doesn’t involve accumulated interest and the rate is only applied to the initial amount only.

General Rule of 72 considerations

The rule assumes that the money will be invested over the time period and that the interest rates won’t change during the holding period. It also assumes that the money will be left to compound over the whole period without any interruptions.

Further, it assumes only the initial amount is invested and that there are no subsequent investments on top of the initial capital.

The rule of 72’s formula works for whole numbers as well as fractions or numbers with decimals. However, the interest rate is added as a whole number and not a decimal!

For example, for an investment earning 8%, the formula for rule of 72 requires you to add it as the whole number 8 and not 0.08! Adding it as 0.08 would give a very crazy answer of 900 years!

72/8 = 9 years

The rule works for anything that grows or decays at a compounded rate such as inflation, GDP, loans, investments such as index funds, money market funds and so on.

How to use the Rule of 72 chart

Here is a handy chart to know when your investment will double in value.

Check out the section below before using the chart for any rates that are not within the 6% to 10% range.

Limitations of the Rule of 72

The rule of 72 is a simple formula to estimate the doubling time given specific interest rates. It gives more accurate results at 8% or any interest rates higher or lower by less than three points.

This simplified formula is less accurate for any interest rates higher or lower than 8% by more than three percentage points.

Therefore, to ensure more accurate results, the adjusted rule requires adding or subtracting 1 from 72 for each time the interest rate diverges from 8% by three percentage points.

Higher rates

Let’s consider a three percentage point increase to a rate of 11%. This would mean an adjusted rule of 73 (72+1). That is (11%-8%) divided by 3 percentage points which is 1.

Therefore, the more accurate doubling time would be 6.64 years.

73/11 = 6.64 actual years to double your investments

Let’s look at the adjustment at even higher rates such as an investment giving an annual return of 23%.

In such a case, the rule would need to be adjusted by (23%-8%) divided by 3 percentage points which is 5. Hence it would become the rule of 77 (72+5) for a more accurate estimate.

In such a case the more accurate doubling time would be 3.35 years.

77/23 = 3.35 actual years to double your investments

Lower rates

What about at lower interest rates? Assume an investment is giving you a rate of 5%. That’s (8%-5%) divided by 3 percentage points which is 1.

Hence it would become the rule of 71 (72-1). In such a case the more accurate doubling time would be 14.2 years.

71/5 = 14.2 years it will take to double your investments

Exact doubling time of an investment

As you have seen in the previous section, the rule of 72 is not as accurate beyond certain ranges.

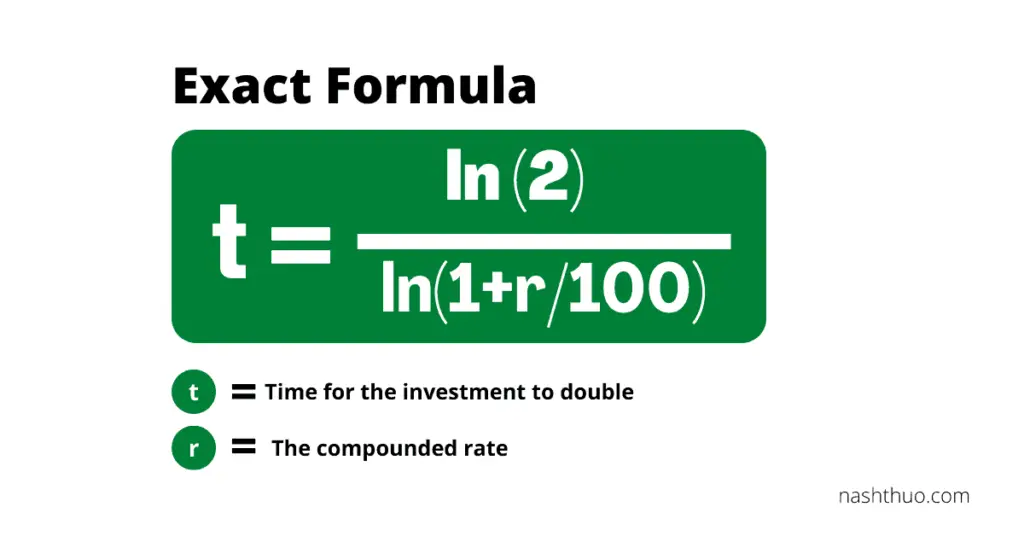
If you have a bit more time, you can use the exact formula recognized by the Mathematical association:

t = ln(2) / ln (1 + (r / 100))

where t is the time for the amount to double;

where ln is the natural log; and

r is the compounded rate.

Let’s work through the formula above assuming we are investing at a rate of 8%.

ln (2) is equals to 0.69314718056;

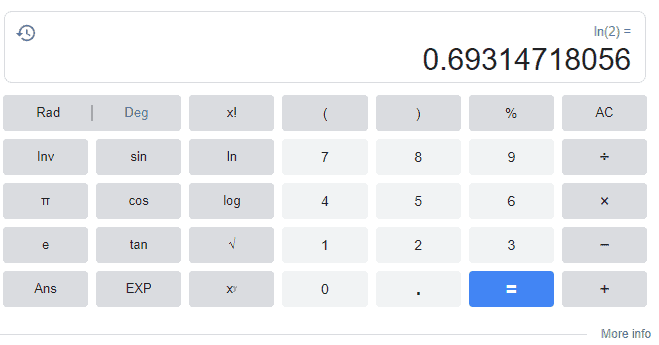
r/100 is 8/100 which is equals to 0.08;

(1 + r/100) is equals to 1.08;

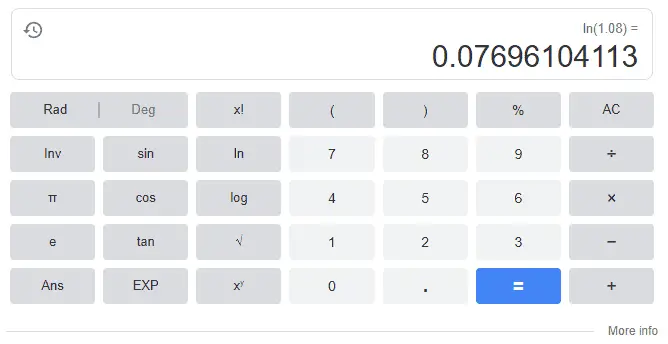
ln (1 + r/100) is ln (1.08) which is equals to 0.07696104113;

Therefore, t = ln(2) / ln (1 + (r / 100) in this case would be 0.69314718056/0.07696104113 which is equals 9.0065 years!

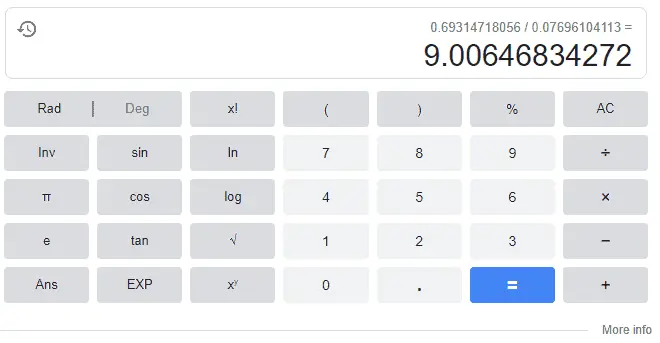
So there you have it! If you are investing at a rate of 8%, the precise doubling time is 9.0065 years compared to the 9 years you would get with the rule of 72 (72/8).

That’s why the latter is preferred as it gives you a fairly accurate but quick answer! However, remember its limitations from the section above which reduce its accuracy beyond the mentioned ranges.

Exact tripling time of an investment

Let’s now learn how to adjust the formula above so as to calculate the exact years it will take for the interest earned to help our investment to triple in value.

We will assume we are investing with the same rate of 8% as in the last section.

The formula remains the same as before:

t = ln(2) / ln (1 + (r / 100))

However, instead of using a log of 2, we will use a log of 3. So the adjusted exact formula would be:

t = ln(3) / ln (1 + (r / 100))

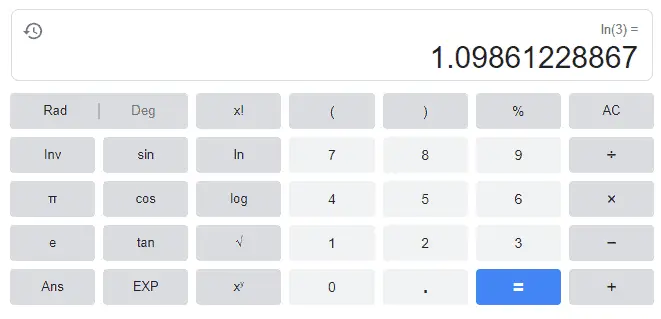
ln (3) is equals to 1.09861228867;

(1 + r/100) is equals to 1.08 as derived in the previous section;

ln (1 + r/100) is ln (1.08) which is equals to 0.0769610411 as derived in the previous section;

Therefore, t = ln(3) / ln (1 + (r / 100) in this case would be 1.09861228867/0.07696104113 which is equals 14.27 years!

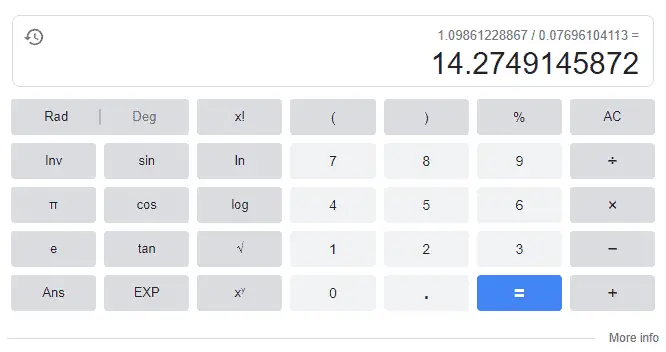
If you are investing at a rate of 8%, the exact tripling time is 14.27 years.

Rule of 115

You can achieve a less accurate estimation but much quicker with the rule of 115 formula below:

115/8 = 14.38 years to triple your investment

Pretty awesome!

Adjustments to the exact formula

You can adjust the log in the formula for any number so as to calculate when your investments will grow by a certain multiple.

I have already covered doubling and tripling effect above. Here is a final illustration to guide the point home.

If you would like to know exactly when your investment will grow by 50% of the initial amount, use the constant in the log as 1.5 rather than 2. Therefore, the adjusted formula in this case would be:

t = ln(1.5) / ln (1 + (r / 100))

You are now a guru on how the exact formula works. Big pat on your back!

You can read more on how the formula was derived in this Stanford University lesson.

Frequently Asked Questions

What is the Rule of 72 in simple terms?

In simple terms, it is a quick way to calculate when your money will double given the rate of return it earns. It assumes that the return is compounded at a fixed rate during the full period.

How does Rule of 72 work?

It works by dividing 72 by the fixed annual return of the investment. The result is the number of years it will take for your investments to double assuming annual compounding.

What is the Rule of 72 and the Rule of 69?

The rule of 72 is useful for fixed annual compounding whereas the rule of 69 is more accurate where there is continuous compounding of the investment.

Continuous compounding is when the frequency of compounding is more than an year. For example daily compounding or monthly compounding and so on.

Whereas the rule of 69 is more accurate, 72 is easily divisible with most numbers hence why the latter is preferred.

Who invented the Rule of 72?

Most people attribute the rule to Luca Pacioli as he referenced it in his 1494 book called Summa de arithmetica. However, it is likely to have been created earlier as Luca refers to it and doesn’t necessarily derive it in his book.